
In this section we begin looking at objects in simple harmonic motion (SHM) . Examples of SHM can be seen around us from pendulums in clocks to a swing moving backwards and forwards.

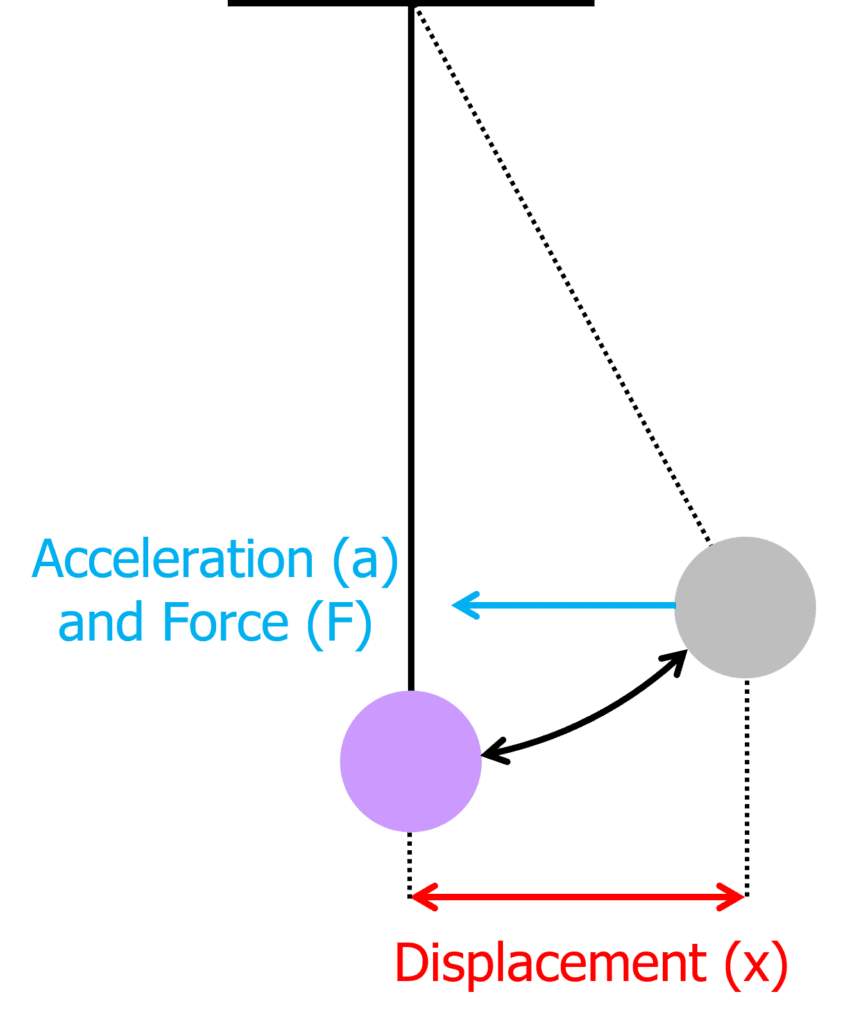

An oscillator is considered to be in simple harmonic motion (SHM) if the acceleration is proportional and opposite in direction to the displacement of the oscillator.
The acceleration of the oscillator always acts in the same direction as the restoring force . This is the force that brings the oscillator back towards the equilibrium position . The acceleration can be calculated using the equation:
The equation shows that the maximum acceleration occurs when the displacement is maximum.
Example: A simple harmonic oscillator has a time period of 2 \text < s>when its maximum displacement is 0.05 \text < m>. Calculate the maximum acceleration.
[3 marks]
The displacement is directly proportional to the negative acceleration of the simple harmonic oscillator. Plotting a displacement-acceleration graph forms a straight line through the origin where the gradient is equal to \omega^2 .

From the graph we can see the points of maximum displacement at either end. These are known as the amplitude of the simple harmonic oscillator (A). The displacement (x) at any given point can be found using the equation:
We can also calculate the speed at any given point of the simple harmonic oscillator. The speed of the oscillator would be at a minimum at its positive and negative amplitudes and at a maximum as it passes the equilibrium where x=0 .
Example: A simple pendulum oscillates with simple harmonic motion with an amplitude of 0.3 \text < m>. The frequency of the oscillations is 5 \text < Hz>. Calculate the speed of the pendulum at a position of 0.1 \text < m>from the equilibrium position.
[3 marks]
A Level AQAProvided a simple harmonic oscillator is undamped , we should expect to see graphs similar to the ones below for any object on simple harmonic motion.
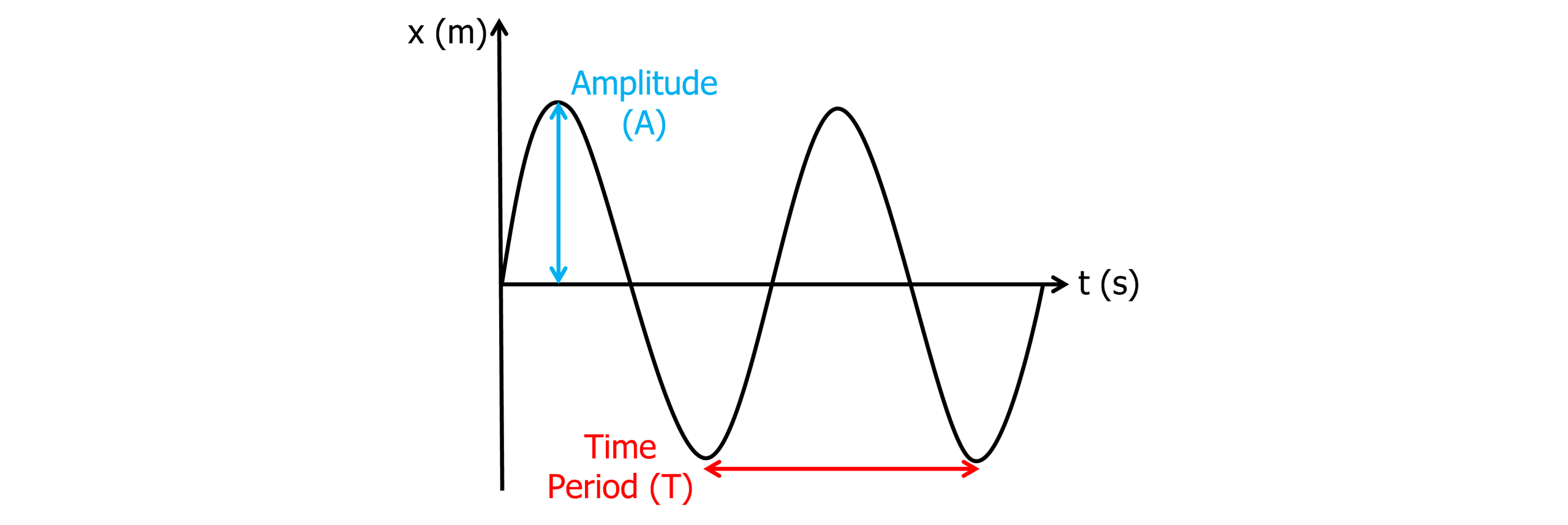
The x-t graph above is a simple sinusoidal graph. The amplitude (maximum displacement) always stays the same as there is no energy lost or gained during oscillations. Calculating the gradient at any point of the displacement-time graph gives the velocity.
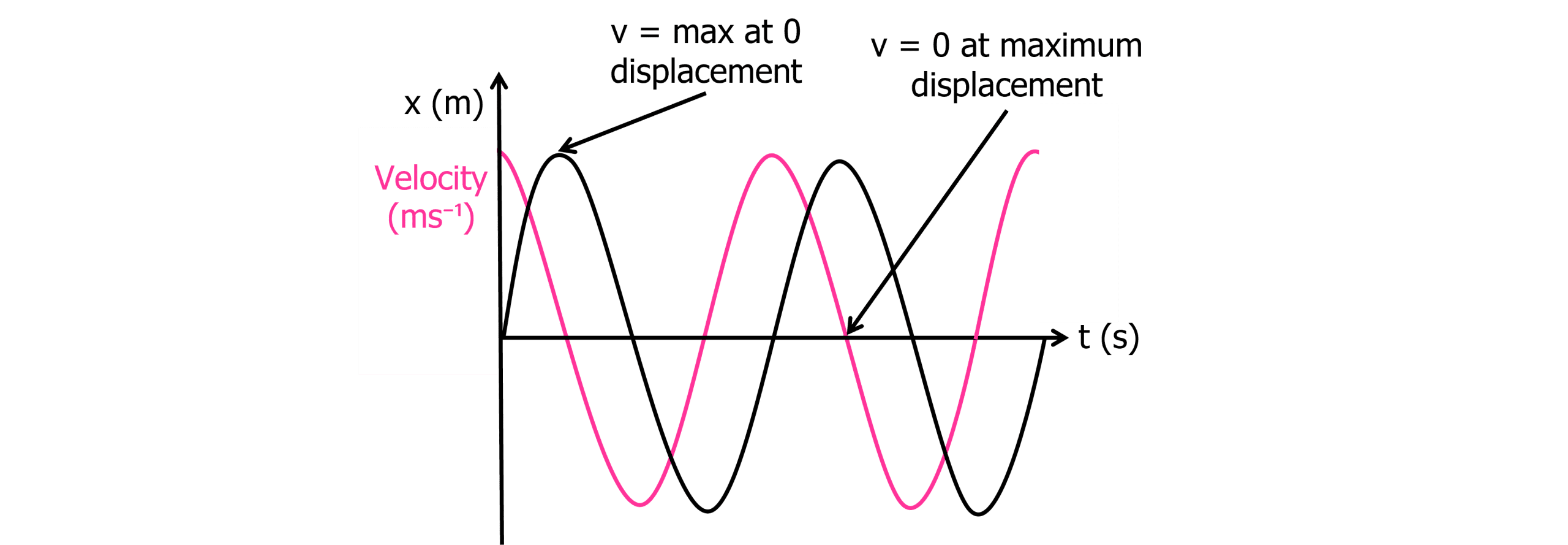
The v-t graph above is a simple cosine graph. When displacement is zero from the previous graph, the velocity is maximum. When the oscillator is at its maximum displacement, the velocity is zero. Again, as no energy is gained or lost, the maximum velocity with each oscillation remains the same. Calculating the gradient at any point on the velocity-time graph gives acceleration.
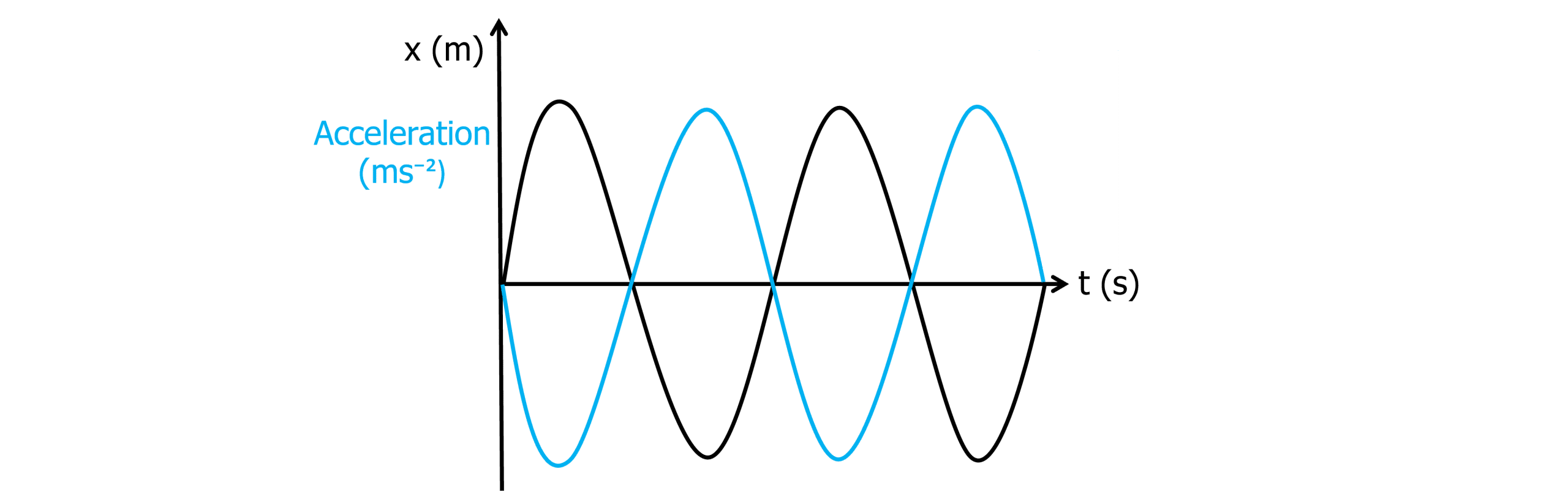
Calculating the maximum velocity of a simple harmonic oscillator can be done using a simpler equation than that learnt previously. As maximum velocity occurs when displacement (x) is zero, the equation can be simplified:
\begin v &= \pm \omega \sqrt \\ &= \pm \omega \sqrt \\ &= \pm \omega \sqrt \end
Calculating maximum acceleration can also be calculated, using the displacement at its maximum. Maximum displacement is known as the amplitude . Therefore the equation changes to:
A Level AQAA mass and a spring can form a system which moves in simple harmonic motion (SHM). If a mass is pulled to maximum displacement on a spring, a restoring force will return the mass to the equilibrium position .

The time period of the mass-spring system can be calculated using the equation:
The equation shows that the time period is proportional to mass and therefore, the greater the mass the greater the time period. It also shows an inversely proportional relationship between time period and spring constant .
Example: A spring with a spring constant of 2.2 \text< Nm>^ is extended by a mass of 5 \text < kg>. The spring is stretched until it moves into simple harmonic motion. Calculate the time period of the oscillation.
[2 marks]
A Level AQA 
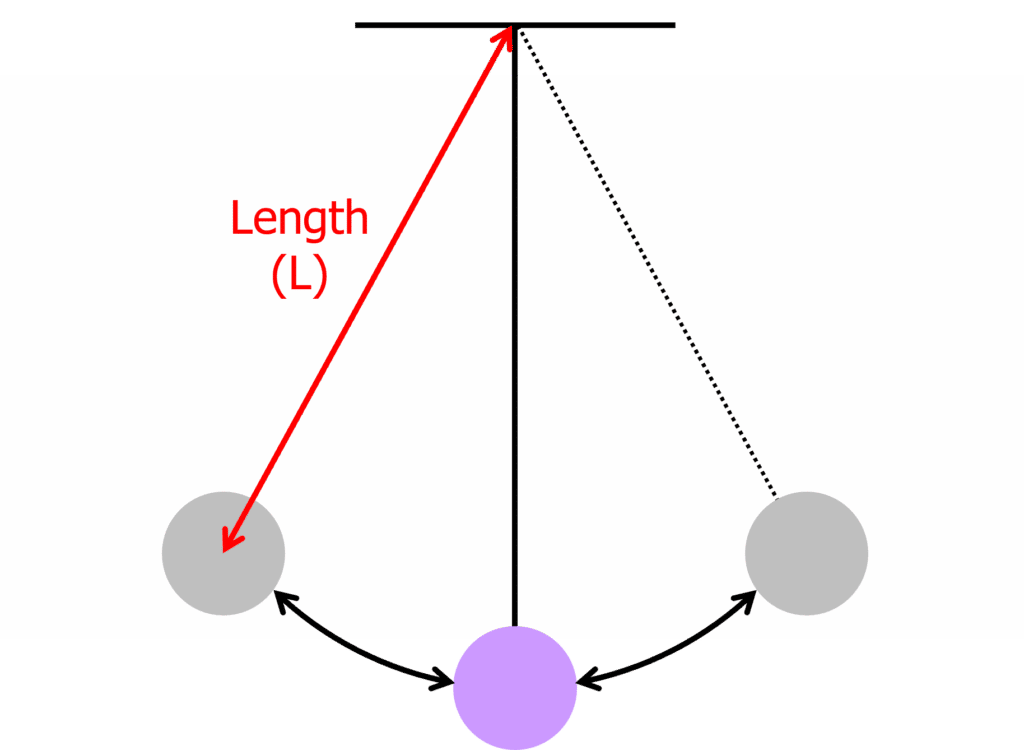

A mass and a string can form a pendulum system which moves in simple harmonic motion (SHM). If a mass is pulled to maximum displacement on a string, a restoring force will return the mass to the equilibrium position. The restoring force is the force responsible for bringing the oscillating object back to the equilibrium position.
The time period of the pendulum can be calculated using the equation:
g is used in the equation above as this represents the restoring force . The equation shows that the time period is proportional to length and therefore, the longer the string the greater the time period.
Example: Calculate the time period of a pendulum of length 0.5 \text < m>on Earth.
[2 marks]